Esophageal pH-impedance monitoring is a tool for diagnosing gastroesophageal reflux in children. The position of the pH catheter is essential for a reliable reading and the current formulas for calculating catheter insertion length are not completely accurate. The aim of the present study was to develop a new formula for adequate insertion of the pH catheter.
Material and methodsA cross-sectional study was conducted on children that underwent pH-impedance monitoring and later radiographic control, to calculate the correct catheter insertion length. The documented variables were age, sex, weight, height, naris to tragus distance, tragus to sternal notch distance, sternal notch to xiphoid process distance, and initial insertion length determined by the Strobel and height interval formulas. A multivariate regression analysis was carried out to predict the final insertion length. Regression ANOVA and Pearson’s adjusted R-squared tests were performed.
ResultsForty-five pH-impedance studies were carried out, 53% of which were in males. The age and weight variables were not normally distributed. In the initial regression model, the variables that did not significantly correlate with the final insertion length were: sex (P 0.124), length determined by the Strobel or height interval formulas (P 0.078), naris to tragus distance (P 0.905), and tragus to sternal notch distance (P 0.404). The final equation: 5.6 + (height in cm * 0.12) + (sternal notch to xiphoid process distance * 0.57) produced an R2 of 0.93 (P 0.000).
ConclusionsThis formula can be considered a valid option for placement of the pH-impedance monitoring catheter in pediatrics.
La pH-impedanciometría esofágica es una herramienta para el diagnóstico de reflujo gastroesofágico en niños, la posición del sensor de pH es crucial para una lectura confiable, las fórmulas existentes para el cálculo de la inserción del catéter no son tan precisas; el objetivo de este estudio fue desarrollar una nueva fórmula para la adecuada inserción de dicho catéter.
Material y métodosEstudio transversal, de niños a los que se realizó pH-impedanciometría y control radiográfico posterior para estimar la longitud de distancia de inserción correcta del catéter; se registraron las variables de: edad, sexo, peso, talla, distancia narina-tragus, tragus-horquilla esternal y horquilla-xifoides así como la longitud inicial de inserción determinada por fórmulas de Strobel e Intervalos de talla. Se realizó un análisis de regresión multivariada para predecir la longitud de inserción final y se obtuvieron ANOVA de la regresión y R cuadrado ajustado de Pearson.
ResultadosSe realizaron 45 pH-impedanciometrías, 53% fueron masculinos. Las variables edad y peso no mostraron distribución normal. En el modelo de regresión inicial las variables sin correlación significativa con la longitud de inserción final fueron: sexo (p 0,124), longitud determinada por Strobel o intervalos de talla (p 0,078), distancia narina-tragus (p 0,905) y distancia tragus-horquilla (p 0,404). La ecuación final: 5,6 + (talla en cm * 0,12) + (distancia horquilla-xifoides * 0,57) alcanza un R2 de 0,93 (p 0,000).
ConclusionesEsta fórmula puede ser considerada como una opción válida para la colocación del catéter de pH-impedanciometría en pediatría.
Combined esophageal multichannel intraluminal impedance and pH monitoring (MII-pH) is increasingly being used in diagnosing gastroesophageal reflux in children.1,2 The main advantage over conventional pH monitoring is its capacity to quantify the frequency and height of the reflux, regardless of the pH, as well as to provide symptom correlation.3 Its main indications include recurrent aspiration pneumonia, unexplained apnea, events similar to nonepileptic seizures, unexplained upper airway inflammation, dental erosion in patients with neurologic involvement, recurrent otitis media, suspected Sandifer syndrome, and previous fundoplication.4 MII-pH can be performed on patients in all age groups. Three commercial devices with their respective software are currently available: Sandhill Scientific (BioView analysis), MMS (Ohmega software), and Vizion (Vizion software). They use flexibles 6 Fr catheters with pH electrodes, multiple impedance rings, and a data-recording device.3 The length of the catheter should be appropriate for the age and height of the patient. Depending on the manufacturer, the distance between the impedance rings and the pH sensor can vary and the pH-measuring electrode can be located in the distal impedance-recording channel or in the second most distal impedance channel. The catheter should be placed transnasally for correct positioning of the pH sensor in the esophagus, which is essential for obtaining reliable pH measurements. The current consensus of the ESPGHAN proposes placing the pH electrode at a distance of 2 vertebral bodies above the diaphragm.1 Some of the formulas proposed for calculating insertion depth are the Strobel formula5 (0.252 × length in centimeters + 5), which is not completely accurate in older children because it overestimates the length of the esophagus; the John Wiley & Sons formula, based on height intervals; and the formula by Mutalib et al., which consists of direct observation through endoscopic placement, in which sensor placement should be calculated at 1.5 cm above the lower esophageal sphincter in infants, at 3 cm in children < 10 years of age, and at 5 cm in children > 10 years of age.6 Formulas also described in the literature are the Hospital de Navarra formula (9.31 + height in cm × 0.197) and the Hospital Infantil Vall d’Hebron de Barcelona formula (9.31 + height in cm × 0.179), among others, based on case series of patients.7 However, fluoroscopic or x-ray control is recommended.8 Even though measuring the naris to tragus distance, tragus to sternal notch distance, and sternal notch to xiphoid process distance has been widely used for calculating the insertion distance of nasogastric catheters, their measurement has not been used for placing pH-impedance catheters. Our experience with the use of the currently existing formulas has shown varying results, therefore, the aim of this work was to develop a new formula for calculating the insertion distance and evaluating its correlation with length measured through radiography.
Material and methodsA cross-sectional study was conducted within the time frame of January 2018 and January 2020. All patients underwent pH-impedance monitoring at the Instituto Nacional de Pediatría in Mexico City during 2019. Sandhill Scientific (BioView analysis) and BS01, BS46, and BS51 catheters were employed. The catheters were introduced transnasally with no sedation, the patients had previously fasted for 4 h, and mechanical restraint was used if the patient was unable to cooperate. Insertion depth was previously calculated using the Strobel or height interval formulas. A control x-ray was carried out on all patients and the necessary adjustments were made to ensure that the pH sensor was located at 2 vertebral bodies above the diaphragm. Patient weight and height (according to standing ability) were obtained, along with the naris to tragus distance, tragus to sternal notch distance, and sternal notch to xiphoid process distance, using a flexible metric tape. The sex of the patient, the initially calculated catheter length, and the final catheter length with the correct position determined through radiography were registered.
Height was measured by the same person, utilizing a Seca® infantometer in children under 2 years of age and a Seca® stadiometer in children over 2 years of age.
The present observational cross-sectional study followed the Strengthening the Reporting of Observational Studies in Epidemiology (STROBE) guidelines.
Statistical analysisThe data were entered into a database, utilizing SPSS version 22 software. The Kolmogorov-Smirnov test for normality was used for the quantitative variables, the sex variable was coded as binary, and median, interquartile range (IQR), mean, standard deviation (SD), frequency, and percentage values were obtained. A multivariate regression analysis was performed to predict the final insertion length, through a step-by-step analysis, removing the independent variables with a p > 0.05. Regression ANOVA and Pearson adjusted R2 tests were carried out, and lastly, the radiographically determined insertion length was compared with each of the existing formulas, using the Wilcoxon test.
Ethical considerationsThe present article was approved by the hospital academic committee and informed consent was requested from the parents and/or patients, depending on patient age.
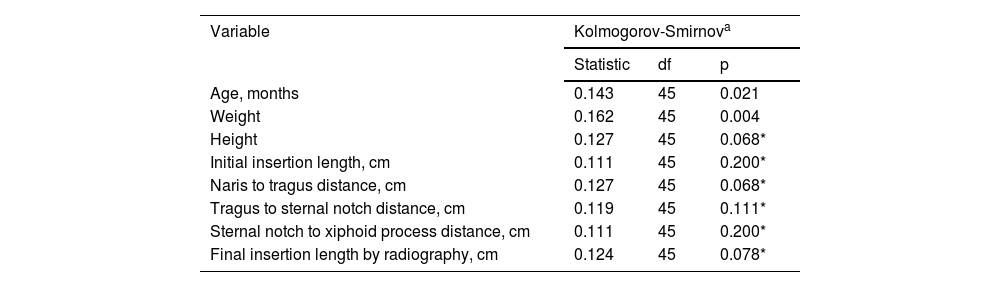
ResultsForty-five pH-impedance studies were carried out. The mean age of the patients was 48 months (IQR: 114), with a minimum age of one month and a maximum age of 210 months. A total of 53% of the patients were males. Median weight was 14 kg (IQR: 22.7), with a minimum weight of 2.75 kg and a maximum weight of 54 kg, and mean height was 101.8 cm (SD: 36.5), with a minimum height of 50 cm and a maximum height of 171 cm. Table 1 describes the normality test results for those variables.
Kolmogorov-Smirnov test for normality carried out on the quantitative study variables
| Variable | Kolmogorov-Smirnova | ||
|---|---|---|---|
| Statistic | df | p | |
| Age, months | 0.143 | 45 | 0.021 |
| Weight | 0.162 | 45 | 0.004 |
| Height | 0.127 | 45 | 0.068* |
| Initial insertion length, cm | 0.111 | 45 | 0.200* |
| Naris to tragus distance, cm | 0.127 | 45 | 0.068* |
| Tragus to sternal notch distance, cm | 0.119 | 45 | 0.111* |
| Sternal notch to xiphoid process distance, cm | 0.111 | 45 | 0.200* |
| Final insertion length by radiography, cm | 0.124 | 45 | 0.078* |
df: degrees of freedom.
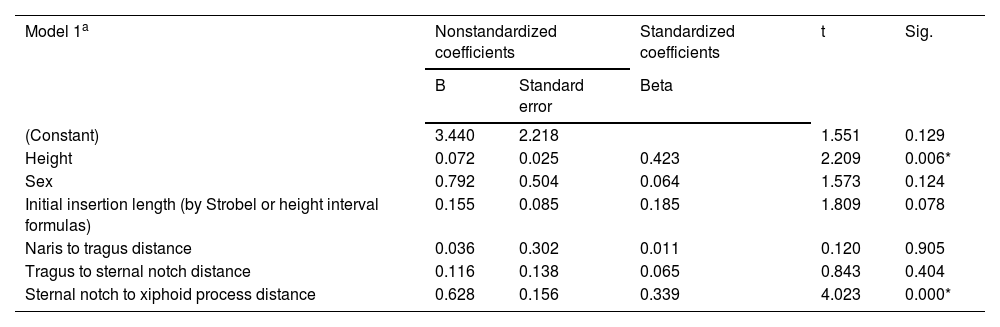
Table 2 shows that the variables of sex, final insertion length (calculated using the Strobel or height interval formulas), naris to tragus distance, and tragus to sternal notch distance were not correlated with the final pH-impedance catheter insertion length with statistical significance. An R2 of 93% was obtained when those variables were excluded from the analysis (Table 3).
Initial model for predicting the pH-impedance catheter insertion length
| Model 1a | Nonstandardized coefficients | Standardized coefficients | t | Sig. | |
|---|---|---|---|---|---|
| B | Standard error | Beta | |||
| (Constant) | 3.440 | 2.218 | 1.551 | 0.129 | |
| Height | 0.072 | 0.025 | 0.423 | 2.209 | 0.006* |
| Sex | 0.792 | 0.504 | 0.064 | 1.573 | 0.124 |
| Initial insertion length (by Strobel or height interval formulas) | 0.155 | 0.085 | 0.185 | 1.809 | 0.078 |
| Naris to tragus distance | 0.036 | 0.302 | 0.011 | 0.120 | 0.905 |
| Tragus to sternal notch distance | 0.116 | 0.138 | 0.065 | 0.843 | 0.404 |
| Sternal notch to xiphoid process distance | 0.628 | 0.156 | 0.339 | 4.023 | 0.000* |
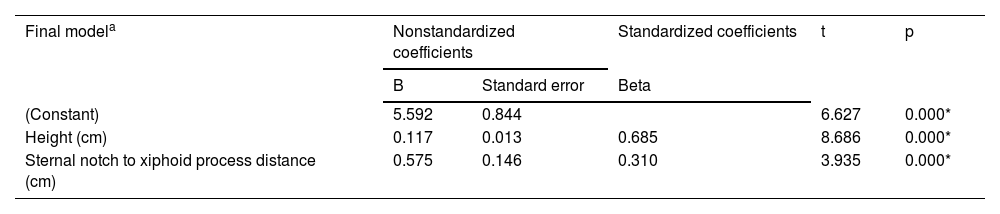
Based on the created model, the final formula for calculating the depth of pH-impedance catheter insertion would be: 5.6 + (height in cm * 0.12) + (sternal notch to xiphoid process distance * 0.57) (Table 4).
Final equation for predicting the pH-impedance catheter insertion length
| Final modela | Nonstandardized coefficients | Standardized coefficients | t | p | |
|---|---|---|---|---|---|
| B | Standard error | Beta | |||
| (Constant) | 5.592 | 0.844 | 6.627 | 0.000* | |
| Height (cm) | 0.117 | 0.013 | 0.685 | 8.686 | 0.000* |
| Sternal notch to xiphoid process distance (cm) | 0.575 | 0.146 | 0.310 | 3.935 | 0.000* |
Upon removing the sternal notch to xiphoid process distance from the model, the R2 slightly decreased (90.5%) but remained statistically significant, with the resulting equation: 7.2 + (height in cm * 0.162).
The comparison between the final catheter insertion length obtained by radiography and each of the pre-existing formulas showed that the Strobel formula, the Navarra formula, and the upper limit of the height interval results had statistically significant differences. The lower limit of the height intervals, as well as the equation proposed by the present authors, showed no significant difference, regarding the final length. The mean difference between the value calculated by the authors’ equation and the final length determined through radiographic control, was 1.2 cm (SD: 1.0) (Table 5).
Comparison of pH-impedance catheter insertion length obtained from radiography versus existing formulas
| Statistics | Final insertion length (cm) | Strobel formula | Navarra formula | Height interval calculation | Authors’ equation | |
|---|---|---|---|---|---|---|
| Lower limit | Upper limit | |||||
| Valid | 45 | 45 | 45 | 41 | 41 | 45 |
| Lost | 0 | 0 | 0 | 4c | 4c | 0 |
| Median (IQR) | 23.0 | 29.7 | 28.6 | 26.0 | 27.5 | 22.6 |
| IQR | 11 | 6 | 14.5 | 12.5 | 12 | 10.9 |
| Wilcoxon test (Z) | −5.712a | −5.712a | −.076a | −4.471a | −.145b | |
| p | 0.000* | 0.000* | 0.940 | 0.000* | 0.885 | |
Table 6 describes the final insertion length compared with the other formulas.
Comparison between the Strobel formula, Navarra formula, the present authors’ formula, and the final length determined through chest x-ray
| Age (months) | Initial insertion length | Strobel | Mean height interval limits | Navarra formula | Present authors’ equation | Final insertion length | |
|---|---|---|---|---|---|---|---|
| 1 | 30 | 17 | 24.83 | 18.75 | 24.81 | 20.54 | 19.5 |
| 2 | 149 | 33 | 40.48 | 33.5 | 37.05 | 31.22 | 29.5 |
| 3 | 10 | 20.3 | 20.37 | 16.25 | 21.33 | 17.32 | 18 |
| 4 | 6 | 18 | 20.12 | 16.25 | 21.13 | 17.21 | 16 |
| 5 | 140 | 35 | 43.81 | 37.5 | 39.65 | 32.06 | 31 |
| 6 | 4 | 17 | 17.85 | 19.36 | 15.58 | 12.5 | |
| 7 | 48 | 29 | 29.7 | 25.5 | 28.62 | 21.42 | 21 |
| 8 | 8 | 20 | 20.12 | 16.25 | 21.13 | 19.1 | 18.5 |
| 9 | 7 | 19.8 | 19.87 | 16.25 | 20.93 | 17.73 | 18 |
| 10 | 210 | 38 | 48.09 | 37.5 | 43 | 37.1 | 38 |
| 11 | 78 | 28 | 32.47 | 26.75 | 30.78 | 23.91 | 24.5 |
| 12 | 79 | 24 | 31.21 | 26.75 | 29.8 | 23.67 | 23 |
| 13 | 138 | 30 | 39.7 | 31 | 36.44 | 28.99 | 26.5 |
| 14 | 13 | 23 | 23.65 | 18.75 | 23.89 | 18.76 | 18 |
| 15 | 43 | 28 | 27.81 | 23.75 | 27.14 | 20.59 | 24 |
| 16 | 1 | 17 | 17.6 | 19.16 | 16.1 | 16.5 | |
| 17 | 63 | 26 | 29.54 | 25.5 | 28.5 | 22.62 | 20 |
| 18 | 8 | 17.5 | 20.12 | 16.25 | 21.13 | 16.58 | 16 |
| 19 | 118 | 30 | 39.52 | 31 | 36.3 | 27.65 | 27 |
| 20 | 47 | 23 | 30.2 | 26.75 | 29.01 | 22.91 | 23 |
| 21 | 32 | 25 | 27.93 | 23.75 | 27.24 | 22.54 | 22 |
| 22 | 163 | 45 | 46.33 | 37.5 | 41.62 | 33.17 | 35 |
| 23 | 3 | 18 | 23.65 | 18.75 | 23.89 | 18.13 | 19 |
| 24 | 87 | 30 | 35.37 | 29 | 33.05 | 25.81 | 27.5 |
| 25 | 3 | 14 | 18.61 | 19.95 | 16.54 | 16 | |
| 26 | 72 | 25 | 33.17 | 26.75 | 31.33 | 22.96 | 24 |
| 27 | 36 | 25 | 36.5 | 29 | 33.94 | 26.95 | 24 |
| 28 | 144 | 34 | 39.27 | 31 | 36.1 | 27.85 | 24 |
| 29 | 20 | 22 | 22.39 | 18.75 | 22.9 | 18.21 | 19 |
| 30 | 3 | 18 | 20.12 | 16.25 | 21.13 | 17.21 | 18 |
| 31 | 12 | 20 | 22.26 | 16.25 | 22.8 | 18.78 | 19.5 |
| 32 | 16 | 15 | 18.1 | 19.55 | 14.43 | 16 | |
| 33 | 37 | 22.5 | 27.93 | 23.75 | 27.24 | 22.54 | 23.5 |
| 34 | 18 | 19 | 22.14 | 16.25 | 22.71 | 18.41 | 21.5 |
| 35 | 115 | 34 | 37 | 29 | 34.33 | 29.06 | 30.5 |
| 36 | 132 | 33 | 41.29 | 33.5 | 37.68 | 29.69 | 31 |
| 37 | 156 | 32 | 42.04 | 33.5 | 38.27 | 31.28 | 34 |
| 38 | 144 | 37 | 45.32 | 37.5 | 40.83 | 35.25 | 37 |
| 39 | 116 | 31 | 38.77 | 31 | 35.71 | 29.21 | 29 |
| 40 | 61 | 26 | 33.1 | 26.75 | 31.28 | 26.08 | 24 |
| 41 | 176 | 30 | 40.03 | 31 | 36.69 | 31.02 | 30 |
| 42 | 8 | 16 | 20.88 | 16.25 | 21.72 | 18.17 | 18 |
| 43 | 173 | 33 | 43.3 | 33.5 | 39.25 | 31.2 | 32 |
| 44 | 59 | 32 | 32 | 26.75 | 30.78 | 24.1 | 24 |
| 45 | 102 | 38 | 38 | 29 | 32.75 | 26.5 | 27 |
There are several formulas in the medical literature for calculating pH-impedance catheter insertion length. Patient height is the calculation parameter taken into account by the majority of them.5,7 However, the existing variability between the length calculated with those formulas and the length obtained through radiographic or manometric control has led other authors to conduct case series to find their own formulas,6 with the aim of avoiding later radiographic or manometric control tests.
Given that other variables, such as patient sex, weight, and age, can influence the length of the esophagus, we decided to include those variables in the present study, without their having shown a significant correlation. Other measurements, such as naris to tragus distance, tragus to sternal notch distance, and sternal notch to xiphoid process distance have been widely used for calculating the insertion length of nasogastric catheters, and so we considered it appropriate to analyze them in the context of pH-impedance monitoring. Nevertheless, only the sternal notch to xiphoid process showed a statistically significant correlation with the final pH-impedance catheter insertion length measured through radiography. The reason the other distances showed no correlation could be explained by the fact that several patients in whom pH-impedance monitoring is indicated could present with anatomic variations of the face or neck, secondary, mainly, to dysmorphic syndromes, making the parameters unreliable.
Our proposed formula, based on height and the sternal notch to xiphoid process distance, correlated well with the radiographically measured length. Therefore, we consider it could be useful for calculating insertion depth. The mean difference between the final radiographic length and the length obtained with our equation was 1.2 cm, which lacks clinical significance if we take into account that the movement of the patient’s head, itself, could move the catheter that same distance; the difference was not above ±4 cm in any of the cases and was below the ±6 cm obtained by Molina.7 Our patient sample ranged in age from one month to 210 months, signifying that it can be extrapolated to a wide pediatric population.
Importantly, pH-impedance measuring is not indicated in all children in whom reflux is suspected. According to the latest guidelines of the NASPGHAN-ESPGHAN for diagnosing gastroesophageal reflux, there is insufficient evidence for supporting the routine use of pH study or pH-impedance monitoring for diagnosing gastroesophageal reflux disease in infants and children, but there are specific indications.9 Therefore, despite the fact that a considerable number of patients with gastroesophageal reflux do not require pH-impedance monitoring, it is essential that when performed, the catheter must be placed with precision, avoiding unnecessary movements of the probe.10
The main limitations of the present study are the sample size, the fact that more studies are needed to validate this formula in the Mexican population and others, and that age-based formulas could not be defined. Therefore, we suggest that future studies include larger samples, to demonstrate the influence of age, and other variables, on insertion length.
ConclusionsOur proposed formula can be used for calculating pH-impedance catheter insertion length in pediatrics. Nevertheless, a chest x-ray still needs to be taken to verify catheter placement. Greater placement precision can aid in manipulating the catheter less and reducing discomfort in the pediatric patient.
Financial disclosureNo financial support was received in relation to this project.
Author contributionsPSC designed the formula and carried out the statistical analysis, as well as drafting the document.
ETM inserted the pH-impedance probes, corroborated the radiographic findings, and also contributed to drafting the document.
FZM, JCL, KIA, EMB, RCB, and JRM reviewed the document, made corrections, and helped with the patients.
Conflict of interestThe authors declare that there is no conflict of interest.
The authors wish to thank the Pediatric Gastroenterology residents for their valuable collaboration and help in the preparation of this formula.